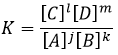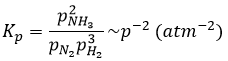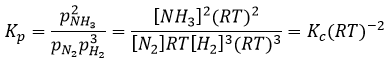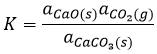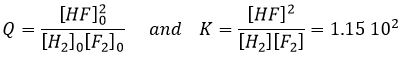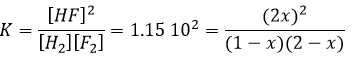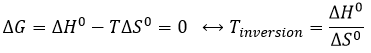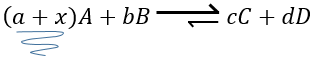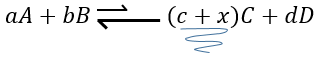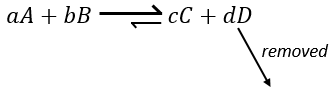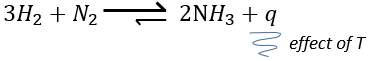Chemical kinetics is the study of the speed of reactions. The speed of reaction is determined by the variation of the quantity of species by unit of time. Usually, we consider the concentrations for species in solution and we consider pressures for gases.
The speed of a reaction is rarely constant and is positive until the system reaches the equilibrium. At the equilibrium, the speed of reaction is zero.
Equilibrium
Given a reaction
The equilibrium is reached when the concentrations of the species don’t vary anymore over time. The constant of equilibrium K, that expresses the law of mass action, is
The constant of the reversed reaction, from right to left, is K’=1/K and if we consider a multiple n of this reaction,
K’’=Kn because stoichiometric coefficients are as exponents in the expression of the equilibrium constant.
The units of K depend of the reaction. For instance, the reaction of Haber that generates ammonia and that we already met before
has an equilibrium constant Kp that depends on the pressures of the reactants and products.
The units of K depend thus on the amount of different products and reactants and on their stoichiometric coefficients. We denoted the constant with a p because we usually consider equilibrium constants in term of concentrations, that we can denote Kc. There is a relation between Kc and Kp that we find from the law of perfect gases pV=nRT
The relation between the pressure and the concentration is thus simply a multiplication by RT. In the expression of K, this RT is also at the power of the stoichiometric coefficient.
For the reaction of Haber, we find
In the case of condensed phases, we won’t consider the concentration nor the pressure because interactions between the species have to be taken into account. We consider the activity of the species instead. The activity reflects the ability of a substrate to react. In condensed phases this ability is decreased by the interactions with the nearby molecules. The activity is proportional to the concentration of a species or to its pressure, multiplied by a coefficient γ. The equilibrium may be heterogeneous if all the species intervening in the reaction are not of the same phase. In this case too, we consider the activities of the species instead of their concentration or pressure. For instance, the reaction
is a reaction where two solids and one gas coexist. It has the following constant of equilibrium:
We choose on purpose a reaction involving solids because their activity is equal to 1. The constant is thus simply proportional to the pressure of CO2. In a closed system, this reaction won’t produce more product if we increase the quantity of reactant. The reaction is only limited by the pressure of the gas.
The reactive quotient Q
To determine the direction of a reaction, we compare the constant of equilibrium K with the reactive quotient Q. Q is determined from the initial concentrations put in presence.
For the reaction
The quotient Q and the equilibrium constant K are respectively
If we consider a system of 5l containing the following quantities of each species:
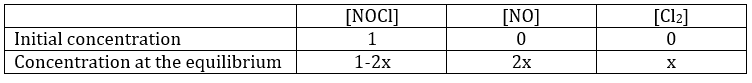
Given that there is no product in this composition, the reaction will obviously go toward the right. It is impossible to obtain negative concentrations. The reaction will proceed until it reaches the equilibrium or that all of one reactant is consumed. We can determine the concentrations of each species at the equilibrium with the following method:
We determine the concentrations of the species. Here we gave the volume of the recipient and the number of moles of each species. We write them in a table and assume that a concentration x will be consumed to reach the equilibrium. The same quantity (in stoichiometric ratios) of product has been formed.

As we know the value of K, we can determine x and thus the concentration of each species.
This equation of the second order gives two solutions but one is negative and thus impossible (no negative concentrations). We can thus determine the concentrations at equilibrium for all the species.
If K is small, for instance in the reaction
has K=1.6 10-5 mol/l, it means that the reaction does not consume a lot of reactants. If we begin the reaction with only 1 mole of NOCl(g), we will consume 0.03mol/l of it.
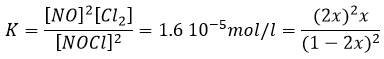
Assuming that x is small, the lower part of the fraction is simplified and
The assumption that 2x<<<1 is correct. A small equilibrium constant also means that the reverse reaction is more favourable (K’=1/K).
Temperature of inversion
The equilibrium depends on the temperature. We have seen in the thermodynamics sections that
At a given temperature, the reactions goes in one direction but if we increase the temperature enough, the reaction will go in the opposite direction. The temperature at which it occurs is the temperature of inversion and is when
If the reaction is performed at p=1atm, we can find this temperature from the tables of ΔH0 and ΔS0:
Modification of the equilibrium
Now, if we consider a system at the equilibrium and we apply a modification on this system, Le Chatelier enounced that the equilibrium will change to counterbalance this modification.
–addition of a substrate: the equilibrium will consume more of this constituent or produce less of it
For instance, let’s consider a reaction at the equilibrium
if we add more A in the system, the equilibrium is displaced to the right to consume some of the added reactant
If we added some C (a product) instead, the equilibrium would have been displaced to the left.
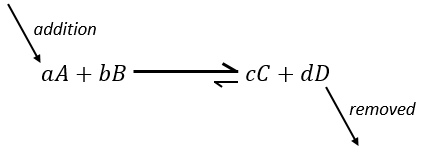
Another way to modify the equilibrium is to remove the products out of the system to displace the equilibrium to the right.
To optimise the speed of a reaction, we can thus add reactants and remove the products continuously.
–temperature: the equilibrium depends on the temperature, as we have seen above. The effect will be different for an exothermic reaction or an endothermic reaction. Indeed,
The variation of temperature affects the exponential with ΔH0. If ΔH0<0, i.e. it is an exothermic reaction, K decreases as result of an increase of temperature and the reaction is thus less efficient. During an exothermic reaction, heat is a product of the reaction
and the temperature increases the heat so the equilibrium is displaced to the left. If the reaction is endothermic (the reverse reaction for instance), an increases of temperature helps the reaction to proceed (more heat is available to be absorbed). The equilibrium is thus displaced to the right.
–pressure/volume: during reactions involving gases, a diminution of the volume will displace the equilibrium toward the side where there is less moles of gas. In the previous reaction, the equilibrium would be displaced toward the right because we generate 2 moles of gas from 4. We can also displace the equilibrium by the addition of an inert gas that will increase the pressure without any modification on the chemical reaction.
Experimental determination of K, ΔH0 and ΔS0
We know that the equilibrium is displaced by a modification of temperature. Imagine that we want to know the values of K, ΔH0 and ΔS0 of a dissolution process like
We know that if there is no Ag+ or Cl– in the solution before the reaction, their concentration will be equal as one of each ion is produced during the reaction. As solids have an activity a=1, the constant of dissociation Ks is
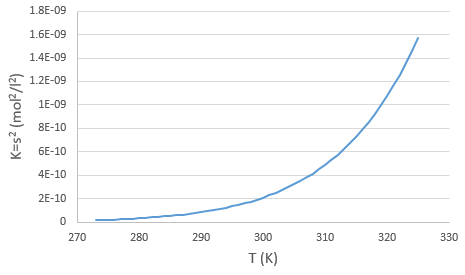
The concentration in ions is thus equal to the square root of the dissociation constant. At a given temperature, we can thus measure the concentration at the equilibrium of one of the ions to determine the value of K. We can repeat this experiment at several temperatures and plot the relation between the temperature T and K.
We see that the dissociation constant Ks increases with the temperature, meaning that if we increase the temperature, we can dissolve more AgCl in solution. AgCl is not very soluble in water: only mg of it per litre will dissolve (the square root of Ks gives the concentration). But we can find more information from this relation. We know that the equation connecting K and T:
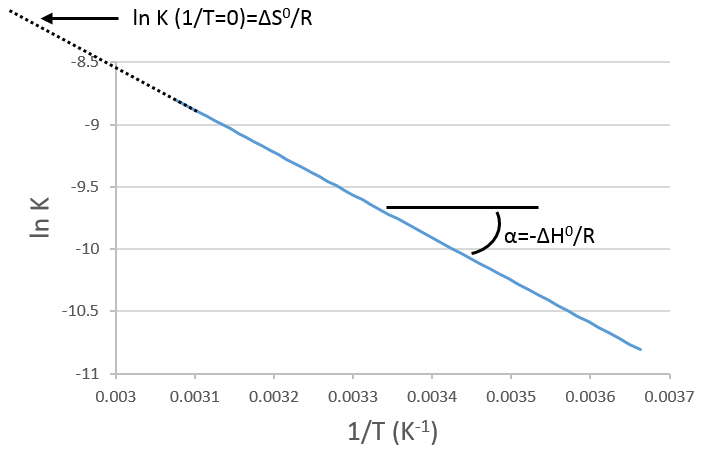
If we plot ln K in function of 1/T, we find a straight line the slope of which is –ΔH0/R.
The intersection with the axis at 1/T=0 will give us ΔS0/R. On the figure, the axis is not at 1/T=0 and the intersection of the curve with the axis doesn’t mean anything.
In the living, ΔG is usually very close to zero so that a stimulus can allow a reaction to take place. In our body, several reactions that would normally not work are coupled with the phosphorous removal of an ATP to allow the process to take place. The ATP is a reserve of energy of our body.