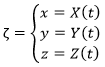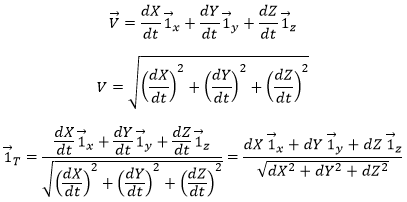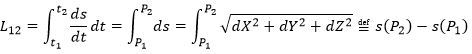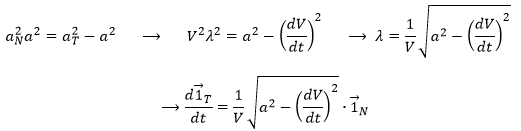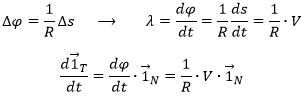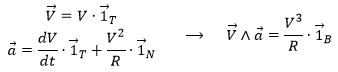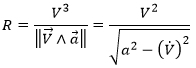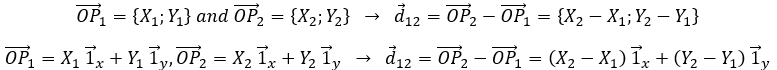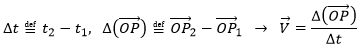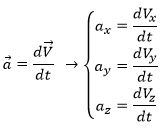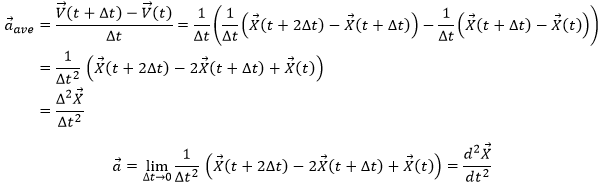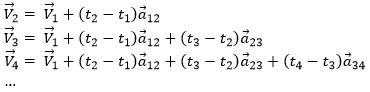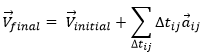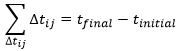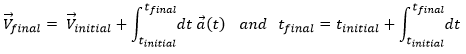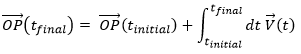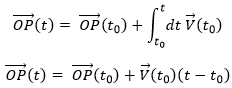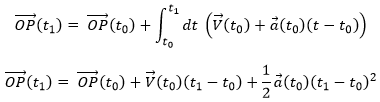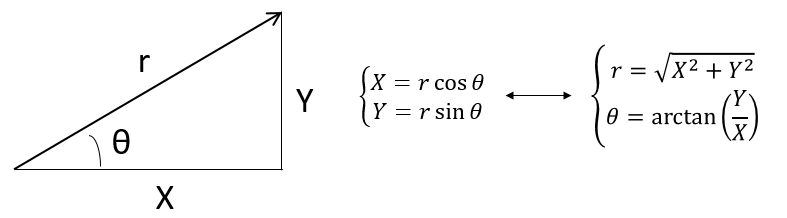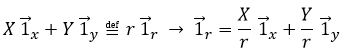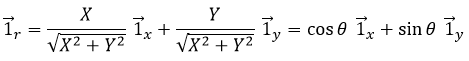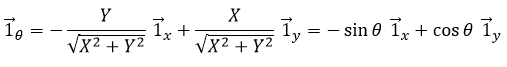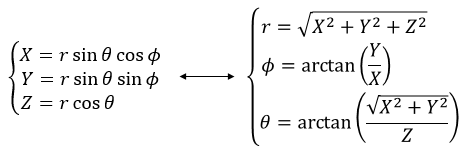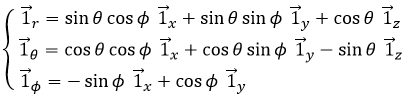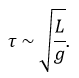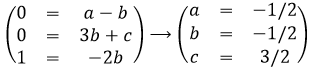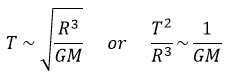Physics

Abstraction made of the time, the geometry is preponderant. In this section, we will discuss a lot about vectors and we define the tangent, the normal and the binormal.

The tangent

The trajectory is the path a moving object follows through space. Let’s analyse this curve ζ from the geometric point of view. The trajectory curve can be defined by

If we take two consecutive positions,  and , the average speed is the vector carried by the rope  The instantaneous speed on her side is a vector tangent to the trajectory.

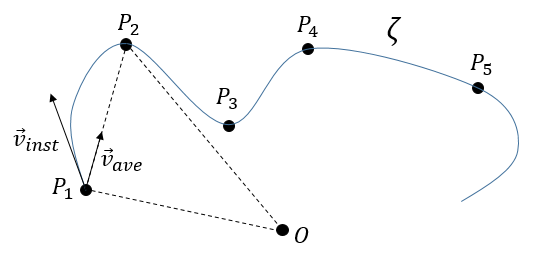
The direction of the speed vector defined thus the tangent to the trajectory. We can get rid of the length of the speed vector and keep its direction by dividing it by its intensity. As a result, we obtain a vector of length 1 tangent to the trajectory.

In the Cartesian system, it corresponds to

In this last expression, the time is not explicitly present. If we defined ds as the instantaneous displacement  we can write

 

The length L of the trajectory is the sum of the lengths of the average displacements and, in the limit, to the integration of the lengths of the instantaneous displacements.

The length of the trajectory s is a parameter intrinsically related to the curve ζ as it doesn’t depend upon the choice of the origin point O, nor of the parameterisation of the curve ζ. It shows, in its infinitesimal version ds, the distance to make on the tangent of the trajectory.

The normal

The vector  being defined, how do we express the geometric aspect related to the acceleration? By construction, the speed vector is tangent to the trajectory of the position vector. The acceleration vector is thus also tangent to the trajectory of the speed vector. How do we represent that in the space?

Let’s derivate the speed vector, giving explicitly its norm V and the direction :

The first term is proportional to  and is thus parallel to the speed vector. The second term is necessarily perpendicular to it. Indeed, the trajectory of  cannot be anywhere else than on a sphere because, by definition, its length does not change. As its derivative is tangent to this trajectory, it is thus tangent to the sphere and thus perpendicular to the radius of the sphere, i.e. to the vector .

We can define the normal vector  as the derivative of the tangent vector.

λ is still to be determined.

• Algebraically

The acceleration  is thus the sum of one tangent part  and one normal part , perpendicular to the first part.

The length of the square of the acceleration is thus given by (the vector product of two perpendicular vectors gives zero)

And then

• Geometrically

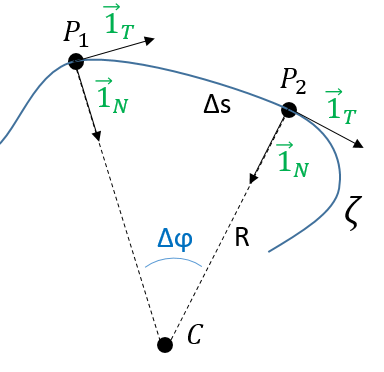
Given two consecutive positions of the point P on the trajectory ζ:  and , and the direction of the speed  and  at the corresponding positions. The tangent unit vector , by definition, doesn’t change its length. However, its direction changes: it turns by an angle .

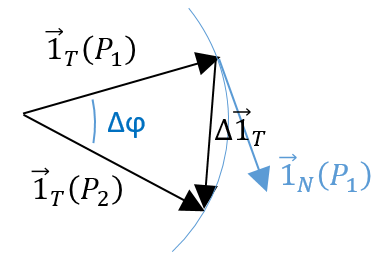
The two tangent vectors delimitate a plane P in which is also their variation . With these three vectors, we can build an isosceles triangle and thus evaluate the length of : 2 sin(/2) and its angle with : π/2-/2.

At the limit, when P₂ tends towards P₁, the length of  becomes  and the angle becomes thus π/2, i.e.  becomes perpendicular to . As a result we can write

λ corresponds thus to the angular speed of rotation of the tangent vector. To be more accurate, we see that if we extend the normal in P₁ and in P₂, they meet at the point C, and that this point C is, at the limit of , the centrum of the tangent circle of the curve ζ in P₁ and P₂. The angle  equals the length of the arc ∆s divided by the radius R of the circle. As a result,

The radius R is called the curvature radius of the trajectory ζ at a given point. 1/R is called the curvature of ζ and the limit plane P formed by the tangent vector and the normal is called the osculating plane of the trajectory ζ.

The binormal

As the tangent  and the normal  are defined, we can build a vector perpendicular to those two vectors: the binormal . To do so, we make the vector product:

This vector is perpendicular to the plane P of the trajectory and is oriented so that the three vectors  can be overimposed to the three Cartesian vectors . Knowing the definitions of the speed and of the acceleration, we also can say that

What allow us to determine the equation of the curvature radius R

The dot over a variable means that it is its derivative over the time. Two dots will mean the second derivative, etc. The readability of the equation can be eased this way. In terms of components of the speed and of the acceleration, we have

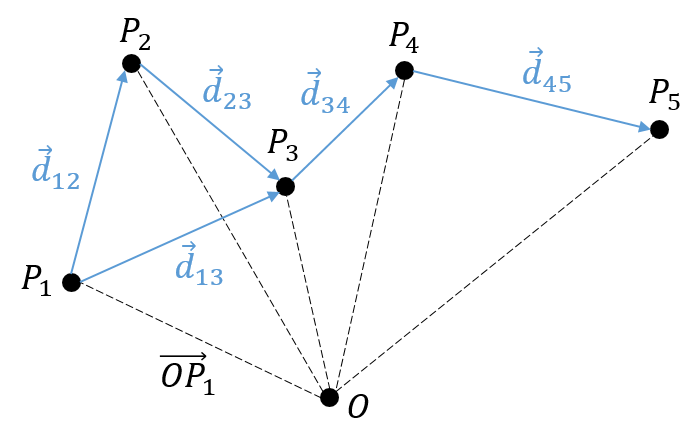
There is a motion if the vector  changes over time. We can thus note this dependence . The variation of position is thus the motion. Noting the position of the object P₁, P₂, P₃, … at consecutive times t₁, t₂, t₃, … , we can introduce the displacement vector  as the vector , and if the objects continues to move, , etc.

Several observations can be made at this point:

• the position  is the addition of the previous position  with the displacement .

• the displacement  equals the sum of the displacements  and .

• it may look obvious, but there is always one position more than there are displacements. As a result, it is impossible to determine the position of the object from its displacements alone. We will need at least one of its positions.

We introduced here the addition operator + on vectors. In the Cartesian system, it is easy to see that the addition and the subtraction operators are simply the mathematical + and – operators applied on the numbers corresponding to the coordinates.

The average speed

Now that the displacements are defined, we can consider that it doesn’t take the same time to make the displacement between P₁ and P₅ directly or with the intermediate steps. The average speed is defined as the displacement by unit of time. At the initial time t₁, the object was at the position P₁ defined by the position vector . At the time t₂, the particle has moved from this position to the position P₂, defined by the position vector . The average speed between these two positions is thus the displacement of the object  divided by the interval of time t₂-t₁.

The speed is said to be average because it depends upon the two chosen positions. One common notation in mathematics is to write differences of two values by the sign Δ. We can thus write

That we can also write, with the explicit dependence over the time:

The instantaneous speed

One concept is now to get rid of the two positions to define an instantaneous speed, associated to a given position and a given time. To do so, we will bring the second point closer from the first and see what happens. We can’t only chose one point because the division by zero is not allowed. However, if this ratio continues to exist when the two points are almost identical, then the instantaneous speed is this value.

It is also the definition of the derivative of the position  with regards to the time, calculated at t₁. The limit towards zero of Δ will be noted d to obtain the notation corresponding to the usual derivative.

To indicate at which time we look for the speed, we use the notation

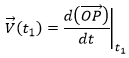
Each component of the speed is given by the derivative of the corresponding component of the position with regards to the time.

The acceleration

The same way we defined the speed as the variation of the position over time, the acceleration a is the variation of speed over time. We define an average acceleration as

and the instantaneous acceleration as the derivative of the speed vector with regards to the time.

The acceleration can be obtained directly from the position by taking its second derivative. We introduce the notations

Note the difference of position of the square exponent: it is the derivative d/dt that is taken twice, not the object of the derivative. It is more evident if we use the definition of the derivative explicitly:

From acceleration to speed

As the acceleration is a difference of speed, there is thus always one acceleration less than there are speeds. From a speed at a time t₁, on can determine the speed at a time t₂ if we are given the acceleration during the interval of time.

From point to point, we can thus determine the speed:

We can generalise this as

Obviously, the sum of the intervals of times equals the elapsed time:

At the limit of the discrete time jumps, the sum becomes an integration

From the speed to the position

We can apply the same method on the speed to obtain the position:

Let insist on the physical meaning of this:

• the integration∫ is a sum,
• this sum is always made on a product,
• this product always contain an infinitesimal difference (here the time dt) and one finite quantity (here the speed V(t)),
• the sum ∫ is defined between a point of beginning and a point of end.

The uniform rectilinear motion

A uniform rectilinear motion (URM) is obtained when the speed of the object doesn’t depend upon time. The speed is thus constant and is defined at the initial time t₀.

As the speed is constant, the acceleration is thus zero.

The equations for the position in each direction correspond to the equation of a straight line in the three dimensions space.

The uniformly accelerated rectilinear motion

Another simple problem is when the acceleration is constant. Similarly to the URM, here we easily find the speed:

And the position:

The first formalism that has to be known in physics is the formalism of the motion. A formalism is associated with a certain rigorous mathematical method, defining symbols and rules that are commonly accepted, in the goal that everybody understands immediately the discussed matter. We will not be interested in the prediction of the motion nor its cause, but in its description alone.

In this section, we consider that every object can be considered as a dot without volume.

The position

The motionis the history of the position of an object, the succession of the positions of this object over the time. To define the position of the object, we need a reference system in which we can give the position: coordinates. Several systems of reference exist and there are several ways to calculate the coordinates of objects. All of them are corrects but some are more convenient than the others. During a trip, you won’t give your position with regard to the sun, the same is true in physics.

Several coordinates are generally necessary to determine the exact position of one object. If you say that you are 10km from Paris, you give an information on your position but we are lacking at least one coordinate to determine your position. Usually you need one coordinate by dimension of the system.

One dimension

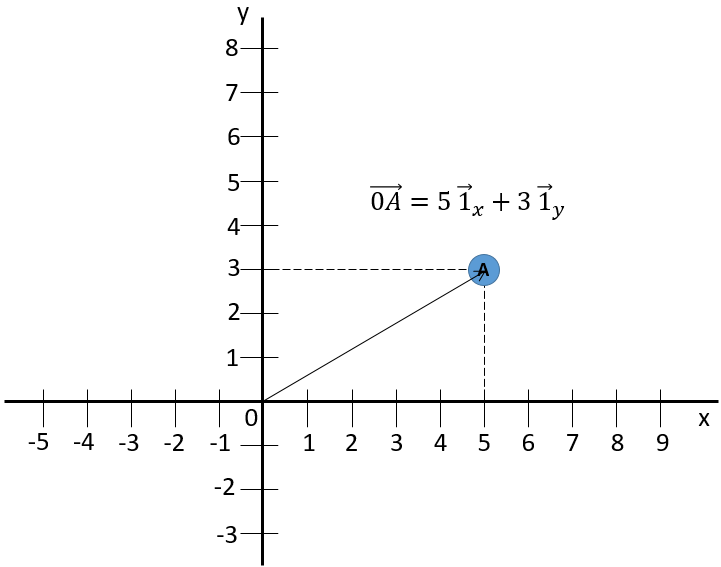
We choose one origin to the coordinate system, the zero point. It is convenient to choose the initial position of the object A as the origin but it is not mandatory. Next we choose a direction that will be the positive positions. In the opposite direction we have the negative positions. Still for convenience, the positive positions are placed in the direction we guess the object will move towards. Imagine that the object moves towards another object B placed at a distance d. The position vector indicates the distance between an object and the origin, and points towards the object with an arrow. The symbol for vectors is topped by an arrow pointing to the right. The position vector for B is

Where  is the unit vector in the direction x (the single direction in this problem).

Two dimensions

The second coordinate is usually orthogonal, perpendicular to the first coordinate to avoid a maximum of angle problems and to benefit the simplicity of the calculation for right triangles. Each coordinate has a direction.

The position vector is now defined by two components from which we can calculate its length if desired.

The addition sign between the two unit vectors is seen as “followed by” and not like the addition of two usual numbers. Another way to write coordinates is to put them in brackets. If we do this, then we don’t write the unit vectors.

This system of reference, the Cartesian system, is not the single one that can be used at two dimensions to determine the position of an object. We can also position the object from its distance r to the origin point and an angle θ from one axis. This reference system is called the polar system.

It is possible to determine the relation between the coordinates X;Y in the Cartesian system and r;θ in the polar system using the relation defining the cosine, the sinus and the tangent:

The unity vector  can thus be calculated.

or

We can also define the unit vector :

Both polar unity vectors depend thus upon θ that should thus be chosen conscientiously.

Three dimensions

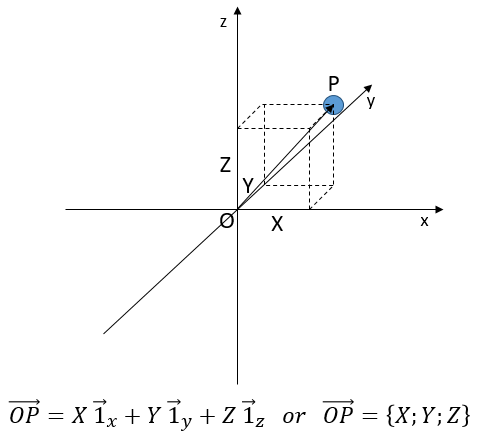
A third coordinate is added in the reference systems. In the Cartesian system, we add a coordinate that is orthogonal to the two previous ones.

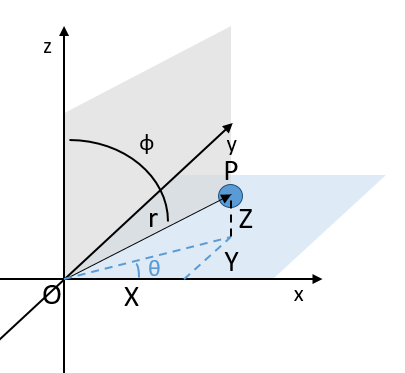
In the polar system, we need a second angle to determine the position of an object. We take the first from the axis x in the xy plane and the second angle is taken from the z axis in the zr plane of the object.

Again, the Cartesian system can be associated to the polar system.

The unit vectors are defined as

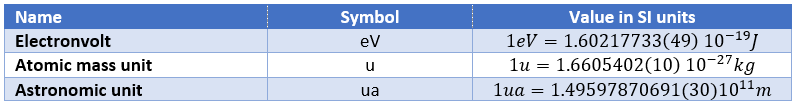
Units are necessary to evaluate quantities. Obviously, a rhinoceros is heavier than a dog, but to know by how much, we need some reference units. Moreover, rhinoceroses don’t all have the same weight. We are thus in need of a consistent unit to measure the weight of objects. The same problem appears for all the possible measures: we won’t measure with precision a distance with regards to the length of bananas because bananas don’t have a consistent length, and it is not convenient for many countries to use this unit of measure. Men have thus defined some quantities as references, quantities that are constant no matter the time or the external conditions (if not explicitly described). Note that some inaccurate units of measure are still in use in daily activities when accuracy is not that important. For instance, when cooking, we add x spoons of oil without giving explicitly the required weight or volume.

On the other hand, we can measure a distance in meters or in miles, the weight in grams or in pounds. All these units have a well-defined value and can serve as reference units. While some countries as the UK or the USA use some different units, international conventions defined an international system of units (SI base units). Their biggest advantage is the simple relation between the units of different quantities. In SI, one millilitre of water occupies one cubic centimetre, weighs one gram, and requires one calorie of energy to heat up by one degree centigrade, which is one percent of the difference between its freezing point and its boiling point. In the American system, you will need to make huge calculations to calculate how much energy it takes to boil a room-temperature gallon of water because you can’t directly relate any of those quantities.

The International Bureau of Weights and Measures (French: Bureau international des poids et mesures) is an intergovernmental organization, established to maintain the International System of Units (SI) under the terms of the Metre Convention (Convention du Mètre, May 20^th 1875). The organisation is usually referred to by its French initialism, BIPM. Its role is to

• establish fundamental standards and scales for the measure of main physical quantities and to conserve the international prototypes;
• compare international standards with national standards;
• ensure the coordination of the corresponding techniques of measurement;
• measure and coordinate the measures of the fundamental, physical constants involved in the above activities.

SI Units

The definitions of the reference units are mainly made to give them a well-known and fixed value.

These definitions fix the speed of light c at 299792458 m/s et the permeability of the void μ₀ at 4π 10^-7 H/m exactly. They also sometimes require some precisions. For instance, we point out that the cesium atom is at rest, that the carbon atoms are not connected, are at rest and in their fundamental state.

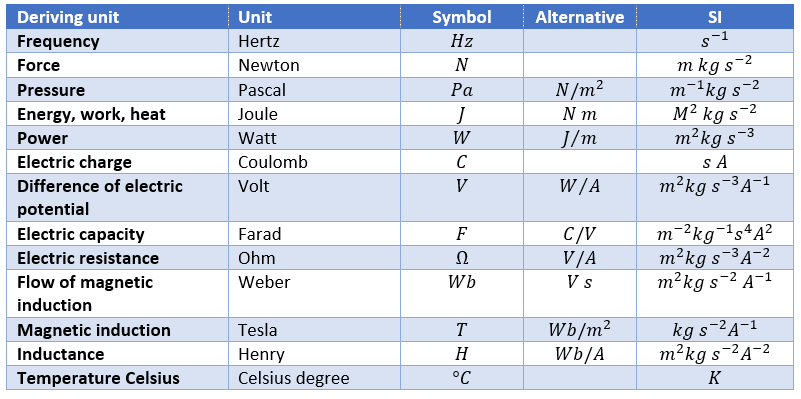
Deriving units

By commodity, some units are the combination of the SI units to express frequently used units.

Finally, there are some quantities without specific unit names

We can point out a few specific units:

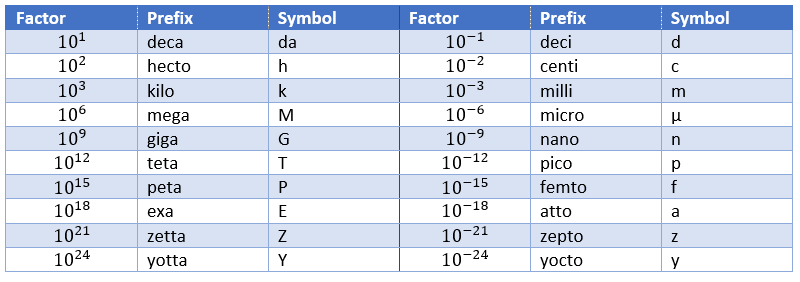
To put an end to this section, we will list the prefix of multiples of the SI units.

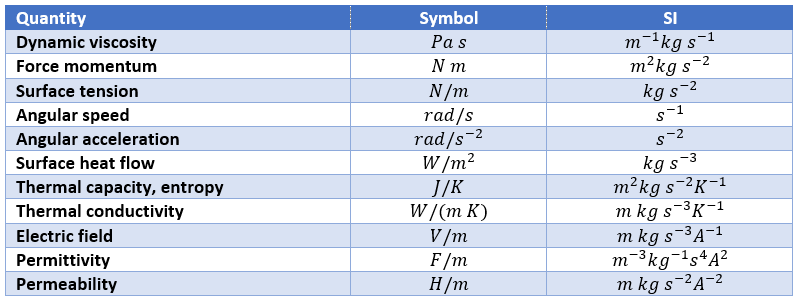
Dimensional analysis

The existence of the IS system means that all the other physical quantities have units that are homogeneous functions of these base units. A function is homogeneous if, making a scale change on all of its variables: x₁ → λ₁x₁, x₂ → λ₂x₂, x₃ → λ₃x₃, … the function itself changes of scale: f(λ₁x₁, λ₂x₂, λ₃x₃,…) = λ₁^α1 λ₂^α2 λ₃^α3…f(x₁, x₂, x₃,…). The units of any quantity, let’s call it Q_r is thus always expressed by a relation like

So if we know the units of one physical quantity, and admitting that there is a relation between this quantity and other variables, and knowing the units of those variables, we can guess the relation between the quantities.

For instance, we observe the swinging of one object attached to one string: a pendulum. The pendulum oscillates because it falls and it is restrained by the string. We want to determine the relation between the times the pendulum takes to make one oscillation, i.e. the period, to the parameters we guess as important: the mass M of the object, the length L of the string, and the gravity acceleration g that affects each object on the planet. The units of the variables are kg for the mass, m for the length, and m/s² for the acceleration. The period is a time and thus its unit is s. The relation between the variables and the period should be something like this:

As there is no kg at the left of the equality, and it is present at the right side of the equation with the exponent a, then we conclude that a=0. Looking at the seconds, their exponent is 1 at the left and -2b at the right, b is thus b=-1/2. Finally, there is no m at the left while it is present at the right side of the equation, thus 0=b+c. As we determined the value of b, we have that c=1/2 and that the global relation is

From our dimensional analysis, we determined that the mass of the object has no influence on the period of the oscillation of the pendulum. Note that we did not write the equal sign: the dimensional analysis doesn’t give the true law; it gives clues on the variables but there can still be numerical factors that can be determined experimentally. On the other hand, the dimensional analysis allows to identify wrong laws not respecting the units of the quantities.

Let’s analyse a second example: the period T of revolution of planets around the Sun. First we identify the important parameters involved in the problem: the mass M of the Sun, the distance R between the planet and the Sun, and a constant G giving the gravitational force. The units of the parameters are respectively kg, m and kg^-1m³s^-2. The period is given in seconds s. The law should be of the form T ~ M^aG^bR^c. For the units, we have the relation

The next step is to identify the exponent of each unit at the left and the right side of the equation:

The law is thus written

This relation is the expression of the Kepler’s law that describes the trajectory of planets of the solar system.

Scales and orders of magnitude

An experimental approach is to estimate the order of magnitude of variables that appear in physical processes. Either we measure the characteristics of one well known property of the matter, or we determine a transition zone between two models of description.

For instance, we can regroup the matter as solids, liquids and gases. One major difference between these three phases is their density, i.e. the mass of the matter for a given volume, given in kg/m³. We can thus regroup liquids as matter with a density with the order of magnitude around 10³kg/m³ (at T=293K, water: 1003kg/m³, olive oil: 910kg/m³, sulfuric acid: 1834kg/m³,…)while gases have a density of order 1 (at T=273K, air: 1.2kg/m³, CO₂: 1.98kg/m³, methane: 0.72kg/m³,…). Between solids and liquids, there is a factor 10 in density (at T=293K: iron: 7893kg/m³, copper: 8954kg/m³, gold: 19320kg/m³,…). We will want to determine the temperature at which a solid becomes liquid, i.e. its melting temperature.

As the interactions between particles of a solid differ from the interactions between particles in a gas, laws are not the same at the microscopic scale than in the astronomic scale, not because the interactions mysteriously disappear, but because we can neglect some interactions. For instance, imagine an interaction between particles that depends directly on the distance between two particles and one interaction that depends on the third power of the distance. If the distance is small, both interactions will have an effect, but as soon as the distance gets large, we can neglect the first interaction.

Definitions and laws

“One of the noblest desire of the man is to know the laws ruling the Universe, and those who contributed to enlighten some of the mysteries were always admired by their peers; they appear as privileged, wearing on them the divine light, a through centuries the generations gaze upon their indelible work and rank them first amongst the glories of the humanity.”

From Achille Cazin (Hachette, 1881).

Physics can be defined as a science that studies the general properties of matter, space, and time, and establishes laws that describe natural phenomena. We will point out the word law first, and ask ourselves if we can clearly, and without ambiguity, define all the objects we are discussing, i.e. space, time and matter. Their definitions are (Oxford dictionaries)

• Space: A continuous area or expanse which is free, available, or unoccupied.
• Matter: Physical substance in general, as distinct from mind and spirit; (in physics) that which occupies space and possesses rest mass, especially as distinct from energy.
• Time: The indefinite continued progress of existence and events in the past, present, and future regarded as a whole.

It is not what we can call clearly defined terms and it doesn’t indicate any of the relations that may exist between them. If we go back in time, Isaac Newton gave its definitions (1687):

• The absolute space, which is without any relation with anything from the surroundings, is always unchanging and immobile. The relative space is any measure or mobile dimension of this space, which is defined with regards to its position with regards to objects that we consider as the immobile space…
• The quantity of matter is the measure that we obtain from its density and its volume…
• The absolute time, true and mathematic, is without relation to anything from the surroundings, and by its nature flows uniformly. The relative time, is any measure, accurate or not, of the duration of an event, that we use in place of the true time. I.e. the hour, day, month,…

It is difficult to avoid cross references and circular definitions. We will abandon the idea to define everything and accept the fact that some notions can make sense without being explicit. Physics is seeking for the laws that rule the reciprocal actions between one object and its surrounding. Enouncing those laws is not an easy job, and we have seen many changes in the history, as the understanding of the scientists evolved. For instance, L. Wouters gives in a school book of 1916 the law for the dilatation of bodies due to heat as

“The first effect that heat produces on bodies is to increase their volume, to dilate them”

This law is based upon its hypothesis on the nature of heat:

“Based on the modern hypothesis on the nature of heat, it results from the vibratory movement of the smallest molecules of the ponderable matter, and is transmitted via a fluid called aether. Aether is a subtle, perfectly elastic, substance that fills the intermolecular spaces as well as the so-called interplanetary voids. Heat is, at the end, a particular state of movement.”

Today, it is obvious that this law is false, mostly because of the notion of aether it is based on. The law itself is true with some exceptions. So, shall we give no definition nor law? Obviously we will, but laws should results from the simple relations obtained from experimentations: if I modify one physical quantity of my experiment, then another physical quantity changes, at this effect is repeated consistently if I repeat the experiment.

To end this introduction, I will extend the citation of Achille Cazin (Hachette, 1881) I opened the introduction with.

“No matter the study on which we work, there are some general rules that one must follow to avoid falling in annoying confusions. …

In physics, we observe all the circumstances around a natural phenomenon; we measure all the available quantities; we seek relations between theses quantities, and these relations are called the law of the phenomenon. When the phenomenon is too complex, and it looks like it is impossible to state one unique law, we modify the phenomenon, we make an experiment. Some circumstances seeming ancillary, are made negligible, and we observe the dominating quantities. From this experiment we obtain an approximate law, and by extension we seek the influence of the neglected circumstances, in which way they alter the law. Doing that, we find the limit law towards which tends the observed law when the circumstances become more and more negligible. Such a law is then used as a fundamental principle, seen as the temporary expression of a physical truth, temporary because one more accurate observation or a new phenomenon can modify the conclusions admitted as truth until now.”